|
|

| Spherical Magnetic Contacts |
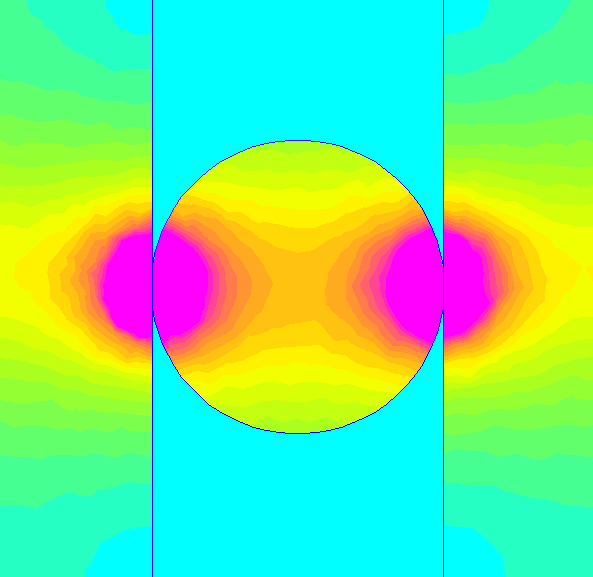
Spherical electrical contacts always weld shut. The reason is purely geometrical. From high school geometry, we all know that a sphere makes a single point contact on any surface. Theoretically, a spherical contact should never work at all. We will show why they make any electrical contact, why they always weld shut, and why it is physically impossible to prevent contact failure by welding within a few closures. A perfect sphere should make a single point contact with zero surface area which is physically unrealizable. There will always be imperfections that yield some contact surface area. We measured a commercially available spherical contact and found the surface contact area, Ac, to be approximately Ac=1*10-12cm2. Very small forces can contribute to this surface area by ball elastic deformation or strain. At 10mA of electrical current, the current density is approximately Jc=1*108A/cm2. Industrial arc welders have typical current densities of Jarc=3.6*104A/cm2. This means that the current density in the measured spherical contact is 2800 times higher than the current density of any industrial arc welder. We life tested a lot of 100 spherical electrical contacts and found 20% welded closed upon first contact. The average statistical failure by welding shut was approximately seven (7) closures. In stark contrast, a reed switch will make contact 1,000,000 closures without any failure by sticking or welding. The test apparatus used a solenoid that created a significant mechanical impulse when it reached the open stop position. It is possible that we would have observed 100% failure upon first contact in the absence of that mechanical impulse breaking the weld loose. There is no known metal or alloy that will not weld at these current densities. Coating with Rhodium or other refractory metals does not mitigate the problem. The current densities are simply too high. Plating with gold only aggravates the problem. It is entirely a geometrical problem. The finite element plot below clearly shows current constriction at extraodinary current densities. Finite element solutions are physically impossible at point contact because the current is divided by zero surface area yielding infinity. Only highly specialized couputers have the resources to deal with surface areas and current densities of actual magnitudes. The surface area in this plot below was 1 billion times larger than the actual switch so that we could realize any kind of solution within the precision of the quad CPU machine with 8GB of RAM. The red areas are current densities around 10 million amperes per cm2. This problem clearly shows disproportionate surface contact relative to the diameter of the sphere. This was necessary due to the small size of the mesh in the vicinity of the contact area needed to solve the problem. |
 |
Use of spherical electrical contacts in high security devices, such as a Balanced Magnetic Switch (BMS), provides false security. The probability that all three spherical electrical contacts will stick closed is very high. This means the device will register closed indicating a secure condition, a false positive, when the door or access panel is open. We know of reports of this happening frequently. The probability of failure by sticking of a glass reed is 1 in 1,000,000, sometimes higher. The probability of a spherical electrical contact is at least 1 in 10 actuations. There have also been claims that spherical contacts are somehow more immune to electrical disturbances such as lightning strikes. This is pure fantasy. The Handbook of Chemistry and Physics 47th Edition has graphs that plot radius of curvature versus electrode separation for arc formation. Gas arc suppressors all use spherical electrodes because they are the optimum shape for arc generation. Any text book on High Voltage devices goes into great detail on this subject. Electrical contacts have been around for over 200 years. One would think that anything as simple as spherical electrical contacts certainly would have been used long before now. This is a perfect example of "Junk Science". As W.C Fields said, " There's a sucker born every minute". |
|